Question

The minute hand of a clock is 5 cm long. What is the linear velocity, in centimeters per minute, at the tip of the minute hand? Round your answer to the nearest hundredth

Answer 1

Answer: .52 is the answer to the nearest hundredth

Explanation:

Ed ge 20 21

Answer 2

The linear velocity is 0.5233 centimeters per minute.

Explanation:

Given : The minute hand of a clock is 5 cm long.

To find : What is the linear velocity, in centimeters per minute, at the tip of the minute hand?

Solution :

The formula to find the linear velocity is
`V=r\omega`

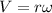
Where,
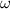`\omega` is the angular velocity

`\omega=(2\pi)/(T)`

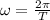
Now, for minute hand, time period is 1 hour.

`1 hr=60\text{ minute}`

`\omega=(2* 3.14)/(60)`

`\omega=(6.28)/(60)`

`\omega=0.104\text{ rad/min}`

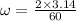
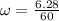
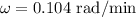
We have given, r=5 cm

Substitute in the formula,

`V=r\omega`

`V=5* 0.104`

`V=0.5233`

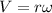
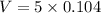
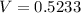
Therefore, The linear velocity is 0.5233 centimeters per minute.