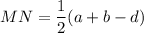Question

Prove: The median of a trapezoid equals half the sum of its bases.

(fill in the blanks of the equation in the second picture with the correct number/letter/sign based off the first picture.)

Answer 1

M(d/2, c/2)
N=((a+b )/, c / 2)
MN= (a +b)/2 - d/2) = (a+b-d)/2
AB=a
CD=b-d
MN=1/2 *(a+b-d)
I think it should be like this

Answer 2

Answer with explanation:

1)

We know that the coordinates of M are:

`M((d)/(2),(c)/(2))`

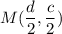
2)

and the coordinates of N are:

`N((a+b)/(2),(c)/(2))`

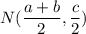
3)

Now, in order to find MN we need to find the length of the line segment MN.

We know that the length of the line segment AB with end points A(a,b) and B(c,d) is given as:

`√((c-a)^2+(b-d)^2)`

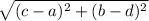
Hence, the length of the line segment MN is:

`=\sqrt{((a+b)/(2)-(d)/(2))^2+((c)/(2)-(c)/(2))^2}`

`=\sqrt{((a+b-d)/(2))^2}\\\\=(a+b-d)/(2)`

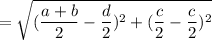
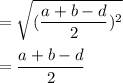
Hence,

`MN=(a+b-d)/(2)`

4)

Now, end points of A are (0,0) and B are (a,0)

Hence, AB is given as:

`AB=√((a-0)^2+(0-0)^2)\\\\Ab=√(a^2)\\\\AB=a`

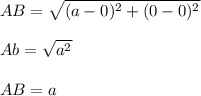
Hence,\

AB=a

5)

Now, CD has end-points as:

C(b,c) and D(d,c)

Hence,

`CD=√((d-b)^2+(c-c)^2)\\\\CD=√((d-b)^2+0)\\\\CD=d-b`

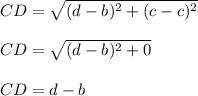
Hence,

CD=d-b

6)

The other form of representing MN is:

`MN=(1)/(2)(a+b-d)`