Answer with explanation:
Here ,let line, x=0 intersect ,segment AB at point M.
So, PM ⊥ AB.
And, AM =BM →→→∵ y axis or segment PM , is Perpendicular bisector of segment AB.
In Δ AMP and ΔB MP
∠AMP = ∠ B MP=90°[→→ Each being 90°]
AM = BM →Line PM , is perpendicular Bisector.
Side MP , is Common.
Δ AMP ≅ ΔB MP→→→[S AS]
So, AP= BP →→[C P C T]
2. We will use distance formula to find AP and BP.
Distance between two points
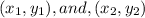 is given by
is given by
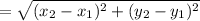
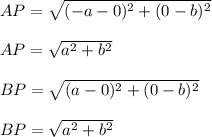
Hence, AP = BP
Where ,Point P, can be located anywhere on y axis.