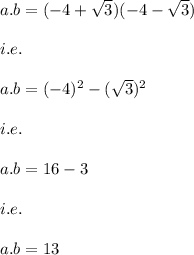Answer:
The exact value of a ⋅ b is:
13
Explanation:
The equation in terms of the variable x is given by:
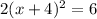
On diving both side of the equation by 2 we get:
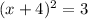
Also,
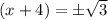
( Since, on taking square root on both the sides of the equation)
Hence, we get:

i.e.

and
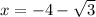
i.e.

Hence,