Question

Graph the solution to the following linear inequality in the coordinate plane.

Answer 1

An inequality on a coordinate plane consist of a boundary line and a area in which each point is the possible solution.

• If the inequality has a sign
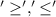  `'\geq' , '\leq'`, then the line will be a solid line and its point are included in the solution.

• If the inequality has a sign '>' , '<' then the line will be a dotted line and its point are not included in the solution.

Given the inequality:
`5x-y>-3`

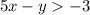
Subtract 5x from both sides we get;

`-y > -5x -3`

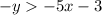
Multiply both sides by -1 we get;

`y < 5x + 3`

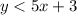
Graph:

Plot the boundary line y = 5x -3 (as a dotted because
`y <` not included equal to) and

the shaded area below as
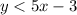`y < 5x -3` as shown below in the graph.

Answer 2

Hello,
Please, see the attached file.
Thanks.