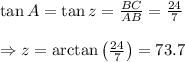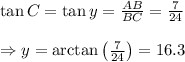Answer:
x = 25
y=16.3
z=73.7
Explanation:
Given :


To find : x, y and z
Solution:
Pythagoras theorem:
In right angled triangle, square of hypotenuse is equal to sum of squares of the remaining two sides .
Here,
 is right angled at B
is right angled at B
On applying pythagoras theorem, we get

Also, we know that
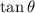 = perpendicular/ Base
= perpendicular/ Base