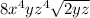Question

Which expression is equivalent to square root 128x^8y^3z^9? Assume y>0 and z>o

Answer 1

It is d because 64 x2 =128

Answer 2

Option (3) is correct.

The given expression
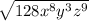`√(128x^8y^3z^9)` becomes
`8x^4yz^4√(2yz)`

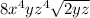
Explanation:

Given : Expression
`√(128x^8y^3z^9)`

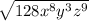
We have to choose an equivalent expression to the given expression
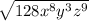`√(128x^8y^3z^9)` and choose the correct from the given options.

Consider the given expression
`√(128x^8y^3z^9)`

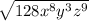
128 can be written as prime factors as 2 × 2 × 2 × 2 × 2 × 2 × 2 =
`2^7`

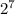
Thus,
`√(128)=√(2^7)=√(2^6\cdot 2)=2^3√(2)=8√(2)`

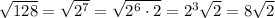
also,
`\mathrm{Apply\:radical\:rule\:}\sqrt[n]{a^m}=a^{(m)/(n)},\:\quad \mathrm{\:assuming\:}a\ge 0`

`√(x^8)=x^{(8)/(2)}`

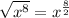
Similarly,
`√(y^3)=√(y^2\cdot y)=y√(y)\\\\ √(z^8)=√(z^8\cdot z)=z^4√(z)`

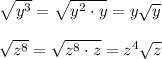
Thus, The given expression
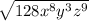`√(128x^8y^3z^9)` becomes
`8x^4yz^4√(2yz)`