Question

Which of the following can be modeled with a linear function? Select all that apply. The cost per visit to a community pool. The neighborhood charges a yearly fee to use the pool. The cost to attend a show at the local theatre. Tickets are $12 each. An endangered species population is decreasing by 15% each year. The distance a bicyclist travels when cycling at a constant speed of 30 mph. The population of a town growing at a constant rate each year.

Answer 1

When you have a linear model, you are adding or subtracting the same amount each time.

The following are linear:

A pool that charges a yearly fee and a per visit fee.

A theater that charges per ticket.

The distance a bike travels at a constant speed.

Answer 2

The options modeling a linear function are 1, 2, 3 and 5.

Explanation:

We know that,

A linear function is of the form
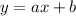`y=ax+b`, where a, b are fixed constants.

Now, according to the options, we have,

1) The cost per visit to a community pool.

Let, x= number of visits to the pool and 'a'= fixed cost of visit.

Then, the cost per visit to the pool y, is
`y=ax`.

So, this represents a linear function.

2) The neighborhood charges a yearly fee to use the pool.

Let, x= number of years and 'a'= fixed fee to use the pool

Then, the yearly fee to use the pool y, is
`y=ax`.

So, this represents a linear function.

3) The cost to attend a show at the local theater. Tickets are $12 each.

Let x= number of shows and the cost of one show is $12.

Then, the cost of 'x' shows y, is
`y=12x`

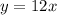
So, this represents a linear function.

4) An endangered species population is decreasing by 15% each year.

Let, a= initial population of the species and x= number of years.

The decrease rate = 15% = 0.15

So, the decrease in the population (y) is given by,

`y=a(1-0.15)^x\\\\y=a(0.85)^x`

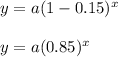
So, this does not represents a linear function.

5) The distance a bicyclist travels when cycling at a constant speed of 30 mph.

Let, x= time and the speed of the cyclist = 30 mph.

So, the distance (y) is given by,

`Distance=Speed* Time\\\\y=30x`

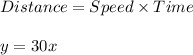
So, this represents a linear function.

6) The population of a town growing at a constant rate each year.

Let, a= initial population of the species and x= number of years and r= constant increase rate per year.

So, the increase in the population (y) is given by
`y=a(1+r)^x`

So, this does not represents a linear function.

Thus, the options modeling a linear function are 1, 2, 3 and 5.