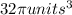Question

The diameter of the base of the cone measures 8 units. The height measures 6 units.

What is the volume of the cone?

24π cubic units
32π cubic units
48π cubic units
64π cubic units

Answer 1

The volume
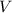`V` of a cone is given by the formula
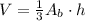`V=(1)/(3)A_b\cdot h` , where
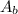`A_b` is the area of the base and
`h` is the height of the cone.

The cone in question here is a right circular base cone. The volume of a right circular base cone with radius
`r` is,

`V=(1)/(3) \pi r^2h\\`.

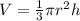In this problem, the diameter is given instead of the radius. If the diameter is 8 units, the radius of the cone is then 4 units because it is half of the radius.

The volume of this cone is therefore,

`V=(1)/(3) \pi r^2=(1)/(3)\pi  (4)^2* 6 =\frac{\pi} {3}* 16* 6= 32\pi .`

The volume of this cone is
`32\pi units^3`

Answer 2

the answer would be 32pi