A linear model of the form
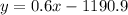 has been established for predicting diabetes among adults. When applied, this model forecasts a 21.5% prevalence of diabetes among adults in 2012. Furthermore, to reach a prevalence of 29.15%, the calculation from the model indicates a specific future year since 2023, based on the linear equation.
has been established for predicting diabetes among adults. When applied, this model forecasts a 21.5% prevalence of diabetes among adults in 2012. Furthermore, to reach a prevalence of 29.15%, the calculation from the model indicates a specific future year since 2023, based on the linear equation.
To find a linear model, we need to find the equation of the line that best fits the given data. A linear model has the form:
 where:
where:
y is the dependent variable (percent of adults with diabetes),
x is the independent variable (year),
m is the slope of the line, and
b is the y-intercept.
We can use the data for two points to find the slope (m) and y-intercept (b). Let's choose the points (2010, 15.1) and (2020, 21.1):

Now, let's calculate m and b:
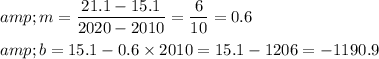
So, the linear model is
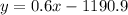
B. To predict the percent of adults with diabetes in 2012 (x=2012), substitute x=2012 into the equation:
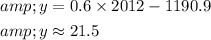
So, the predicted percent in 2012 is approximately 21.5%.
C. To find the year when the model predicts the percent to be 29.15% (y=29.15), substitute y=29.15 into the equation:
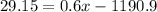
Now, solve for x:

So, the model predicts the percent to be 29.15% around the year 2032.