Question

A generic salt, ab3, has a molar mass of 203 g/mol and a solubility of 8.30 g/l at 25 °c. what is the ksp of this salt at 25 °c?

Answer 1

7.56 × 10⁻⁵

Step-by-step explanation:

Let's consider the solution of the poorly soluble salt AB₃.

AB₃(s) ⇄ A³⁺(aq) + 3 B⁻(aq)

The molar solubility of the salt (S) is:

`(8.30g)/(203g/mol * 1L ) =0.0409 M`

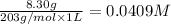
In order to relate S to the solubility product (Ksp), we will use an ICE Chart.

AB₃(s) ⇄ A³⁺(aq) + 3 B⁻(aq)

I 0 0

C +S +3S

E S 3S

The solubility product is:

Ksp = [A³⁺].[B⁻]³ = S . (3S)³ = 27 S⁴ = 27 (0.0409)⁴ = 7.56 × 10⁻⁵

Answer 2

The salt is AB₃ that has a solubility of 8.30 g/L
when the salt dissolves, it dissociates as follows;
AB₃ --> A³⁺ + 3B⁻
The molar solubility is the number of moles that can dissolve in 1 L of solution.
Molar solubility of salt is 8.30 g/L / 203 g/mol = 0.04 mol/L, Solubility of A³⁺ is 0.04 mol/L and solubility of B⁻ is 0.04 x3 = 0.12 mol/L
ksp is the solubility product constant and calculated as follows;
ksp = [A³⁺][B⁻]³
= 0.04 mol/L x (0.12 mol/L)³
= 0.04 x 0.0017
ksp is 6.9 x 10⁻⁵