Question

Two triangles are similar.The the smaller triangle has side 10,20 and 15 units the larger triangles longest side is 52 units.What is the perimeter of the largest triangle?

Answer 1

Answer: The perimeter of the largest triangle = 117 units.

Explanation:

Sides of smaller triangle = 10,20 and 15 units

Longest side = 20 units

Longest side of larger triangle = 52 units

Sides of two similar triangles are proportional.

Let k be proportionality constant.

`k=\frac{\text{Length in larger triangle}}{\text{Length in smaller triangle}}`

`k=(52)/(20)\\\\\Rightarrow\ k=(13)/(5)`

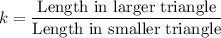
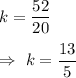
Length of other two sides,

`10*(13)/(5), 15*(13)/(5)\ i.e.\ 26\text{ units}, 39\text{ units.}`

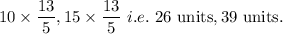
So sides of larger triangle = 52 units, 26 units , 39 units

Perimeter of largest triangle = 52 +26+39 = 117 units

Hence, the perimeter of the largest triangle = 117 units.