Question

Data Table:

Coefficient of friction
(=tan(angle)
Trial #
Length of book
Angle
(=sin(height/length))
Height of end
average
Diagram:
length of book
(block
boek
height of
end
table
Extension Questions:
1. If the coefficient of friction between a wheelchair wheel and concrete was 0.4, what is the
maximum angle the ramp could have?
2. Would it make a difference, when determining the maximum angle of a ramp, if you were to use
the coefficient of kinetic friction rather than static friction? Which would be a better choice?
Justify your answer.
3. What is the purpose of a switch-back when driving up the side of a mountain (a switchback is
when the road zig-zags, reversing direction and appearing to go back the way it came, but at a
higher elevation)?

Answer 1

Hi there!

1.

To find the maximum angle that the ramp can have, we must find the angle at which the net force on the wheelchair wheel along the axis of the plane is still 0 N.

We can do this using a summation of forces. The only two forces acting on the wheelchair wheel in this situation is the force due to STATIC friction and gravity:

⇒ Force due to static friction: Fs = μN

In this case, N = mgcosθ, so :

`F_s = \mu mgcos\theta`

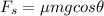
⇒ Force due to gravity along the axis:

`F_g = mgsin\theta`

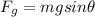
These two must sum up to zero, so:

`\Sigma F = mgsin\theta - \mu mgcos\theta = 0 \\ \\ mgsin\theta = \mu mgcos\theta`
Cancel the mass and gravity:

`sin\theta = \mu cos\theta\\ \\ tan\theta = \mu \\ \\ tan\theta = 0.4\\ \\ tan^(-1)(0.4) = \boxed{21.80^o}`

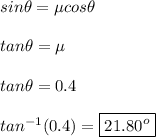
2.

Yes. The coefficient of static friction provides you with the MAXIMUM amount of friction force (resistance) that must be overcome for the object to begin moving, while the coefficient of kinetic friction allows you to find the friction force experienced by a MOVING object. In this instance, the coefficient of KINETIC friction is most appropriate because the wheelchair is moving on the ramp. However, if finding the maximum angle of a ramp with an object placed on it, the coefficient of STATIC friction would need to be used in determining the angle that will cause the object to begin sliding.

3.

A switch-back decreases the angle of inclination that a car must travel, so the risk of the car sliding down is decreased because as the angle DECREASES, the force due to friction INCREASES. Friction is what prevents a car from sliding, so minimizing the angle of a ramp/road will increase the friction force and lower the risk of sliding down.