Question

⎩

⎪
⎨
⎪
⎧
​
h(1)=−75
h(n)=h(n−1)−10
​
Find an explicit formula for h(n)
h(n)=

Answer 1

the explicit formula for h(n) is, -65-10n

Explanation:

Given that:

h(1) = -75

h(n) = h(n-1)-10 ......[1]

Put n =2 in [1] we have;

`h(2) = h(1)-10 = -75-10 = -85`

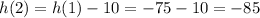
Similarly for n = 3

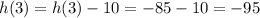`h(3) = h(3)-10 = -85-10 = -95` and so on...

The series we get;

`-75, -85, -95, ....`

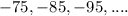
This is an arithmetic sequence series with common difference(d) = -10

Since,

-85-(-75) = -85+75 = -10,

-95-(-85) = -95+85 = -10 and so on

First term(a) = -75

the Explicit formula for arithmetic sequence is given by:

`a_n = a+(n-1)d`

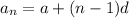
where a is the first term,

d is the common difference and

n is the number of terms.

We have to find the explicit formula for h(n);

`h(n) = a+(n-1)d`

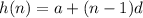
Substitute the given values we have;

`h(n) = -75+(n-1)(-10)`

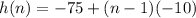
or

`h(n) = -75-10n+10 = -65-10n`

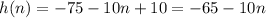
Therefore, the explicit formula for h(n) is, -65-10n