Question

What is the measure of XY?

Answer 1

B. 53

Explanation:

Just did it on A pex

Answer 2

Answer: Option 'B' is correct.

Explanation:

Since we have given that

Measure of UZ = 127°

∠YVZ=∠XVZ=90°

Now, we know that ∠YVZ and ∠UVZ are linear pair.

so, it becomes,

`\angle YVZ+\angle UVZ=180^\circ\\\\90^\circ+\angle UVZ=180^\circ\\\\\angle UVZ=180^\circ-90^\circ\\\\\angle UVZ=90^\circ`

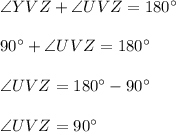
So, now we have the measure of angle 90° and one intercepted arc which is equal to 127°.

As we know that the measure of angle is half the sum of the intercepted arcs,

so, it becomes,

`\angle YVZ=(UZ+XY)/(2)\\\\90^\circ=(UZ+XY)/(2)\\\\90* 2=UZ+XY\\\\180^\circ=127^\circ+XY\\\\180^\circ-127^\circ=XY\\\\XY=53^\circ`

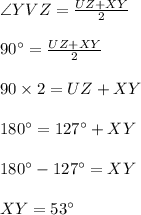
Hence, the measure of XY is 53°.

Therefore, Option 'B' is correct.