Remember that stretching or compressing the graph of a function is a transformation in which you multiply the whole function by a constant

. If
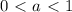
, you are compressing the graph of the function by a factor of

. If

, you are stretching the graph of the function by a factor of

.
1. Here we want to stretch the graph by a factor of 2. Since 2 > 1, we just need to multiply the whole function by 2. Therefore,
A stretch by a factor of 2 for the exponential growth function f(x)= a(9/4) occurs when a =2.
2. Here we want to stretch the graph by a factor of 11/3. Since 11/3 > 1, we just need to multiply the whole function by 11/3. Therefore, a stretch by a factor of 11/3 for the exponential decay function f(x)= a(3/5) occurs when a=11/3.
3. Here we want to compress the graph by a factor of 1/3, so
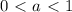 . The only number in our options that satisfy that condition is 1/3. Therefore, a shrink by a factor of 1/3 for the exponential growth function f(x)= a(7)^x occurs when a = 1/3.
. The only number in our options that satisfy that condition is 1/3. Therefore, a shrink by a factor of 1/3 for the exponential growth function f(x)= a(7)^x occurs when a = 1/3.
4. Here we want to compress the graph by a factor of 2/5, so
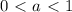 . since 0<2.5<1, we just need to multiply the whole function by 2/5. Therefore, A shrink by a factor of 2/5 for the exponential decay function f(x)= a(2/9)^x occurs when a =2/5.
. since 0<2.5<1, we just need to multiply the whole function by 2/5. Therefore, A shrink by a factor of 2/5 for the exponential decay function f(x)= a(2/9)^x occurs when a =2/5.