2 Answers

Dashesy · Answer 1 · 2019-12-16T08:35:43+0000

Since we know that trapezoid mid-segment theorem states that mid segment of a trapezoid is half the sum of trapezoid's two parallel sides.

We can see that segment AB and CD are parallel. Let us find lengths of segment AB and CD using distance formula.

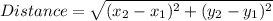
$Distance =\sqrt{(x_2-x_1)^(2) +(y_2-y_1)^(2)$

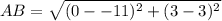
$AB =\sqrt{(0--11)^(2) +(3-3)^(2)$

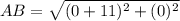
$AB =\sqrt{(0+11)^(2) +(0)^(2)$

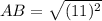
$AB =\sqrt{(11)^(2)$

AB =11

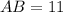
Now let us find side length CD.

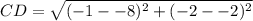
$CD =\sqrt{(-1--8)^(2) +(-2--2)^(2)$

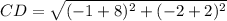
$CD =\sqrt{(-1+8)^(2) +(-2+2)^(2)$

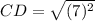
$CD =\sqrt{(7)^(2) +(0)^(2)$

$CD =\sqrt{(7)^(2)$

CD =7

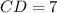
Let us find length of mid segment of our given trapezoid using mid segment theorem.

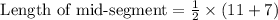
$\text{ Length of mid-segment}=(1)/(2) * (11+7)$

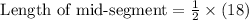
$\text{ Length of mid-segment}=(1)/(2) * (18)$

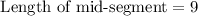
$\text{ Length of mid-segment}=9$

Therefore, length of the mid-segment of our given trapezoid is 9 units.

Please log in or register to add a comment.