Question

If 2x2 – 21x + 27 = (2x – 3)(x – 9), which equation(s) should be solved to find the roots of 2x2 – 21x + 27 = 0? Check all that apply.

A. x – 9 = 0
B. 2x + 3 = 0
C. 2x – 3 = 0
D. x + 9 = 0
E. 2x – 3 = x – 9

Answer 1

both A. x – 9 = 0 and C. 2x – 3 = 0

Answer 2

The equations that should be solved to find the roots of the equation are:

A . x-9 = 0

C. 2x-3 = 0

Explanation:

We are given the factorization of a quadratic polynomial expression in terms of variable " x " as:

`2x^2-21x+27=(2x-3)(x-9)`

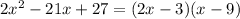
The roots of the equation:

`2x^2-21x+27=0`

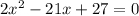
is the roots of the equation:

`(2x-3)(x-9)=0`

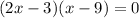
We know that the roots of any equation of the type:

`(x-a)(x-b)=0`

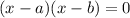
are x=a and x=b

Hence, the correct options are:

A . x-9 = 0

C. 2x-3 = 0