Question

A polynomial function can be written as (x + 2)(x + 3)(x − 5). What are the x-intercepts of the graph of this function?

Answer 1

(-2,0) (-3,0) (5,0)
Make each factor or parentheses equal to zero and solve to get the x value of the intercepts.
x+2 = 0
subtract 2 from both sides
x = -2

x+3 = 0
subtract 3 from both sides
x = -3

x-5 = 0
add 5 to both sides
x = 5

Answer 2

x-intercept of the polynomial is at x=-2,-3 and 5.

Explanation:

Given : A polynomial function can be written as (x + 2)(x + 3)(x − 5).

To find : What are the x-intercepts of the graph of this function?

Solution :

x-intercept is defined as the point where y=0.

To find the x-intercept we have to substitute the polynomial equal to zero.

i.e.
`(x + 2)(x + 3)(x- 5)=0`

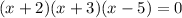
Now, Solve each term one by one,

`(x + 2)=0`

`x=-2`

`(x + 3)=0`

`x=-3`

`(x-5)=0`

`x=5`

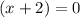
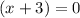
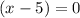
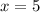
Therefore, x-intercept of the polynomial is at x=-2,-3 and 5.