Question

Use the Law of Sines to find the missing angle of the triangle. Find angle B given that C = 83, A = 44, and angle A = 31.

A. 2.7°

B. 15.8°

C. 164.2°

D. 76.3°

Answer 1

Option D is correct

Explanation:

Sine rule is

`(sinC)/(c)= (sinA)/(a) = (sinB)/(b)`

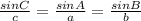
we have c =83 , a =44 And A =31 degrees

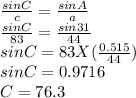`(sinC)/(c) =(sinA)/(a) \\(sinC)/(83) =(sin31)/(44) \\sinC=83X((0.515)/(44) )\\sinC =0.9716\\C =76.3` or 103.3

If C = 76.3 degrees or C =103.3 and A = 31 degrees

Using triangle angle sum property

A+B+C = 180

31+76.3 +B = 180

B=180 -31-76.3

B= 72.7

when C =103.3

then B = 180- 31-103.3

B = 45.7

According to options given ,Its asking for Angle C and which is 76.3

Answer 2

∠C = arcsin(83/44*sin(31°)) = 76.3°

∠B = 180° -31° -76.3° = 72.7° . . . . . . . probably matches selection A

_____
There is another solution:
∠C = 180° -76.3° = 103.7°

∠B = 180° -103.7° -31° = 45.3° . . . . . not an offered choice