Question

A black hole is an object so heavy that neither matter nor even light can escape the influence of its gravitational field. since no light can escape from it, it appears black. suppose a mass approximately the size of the earth's mass 6.42 × 1024 kg is packed into a small uniform sphere of radius r. use: the speed of light c = 2.99792 × 108 m/s. the universal gravitational constant g = 6.67259 × 10−11 n m2 /kg2 . hint: the escape speed must be the speed of light. based on newtonian mechanics, determine the limiting radius r0 when this mass (approximately the size of the earth's mass) becomes a black hole. answer in units of m

Answer 1

We are given information:
M =
`6.42 * 10^(24) kg`
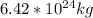
c =
`v_(esc) =`
`2.99792 * 10^(8) m/s`
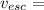
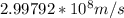
G =
`6.67259 * 10^(-11) N m^(2) / kg^(2)`

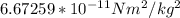
When we have huge gravity such as in black hole Newton mechanics does not apply very well and we need to use theory of relativity. However we can try to calculate needed radius.

Energy conservation law states:

`E_(total) =-E_(potential) +E_(kinetic)`
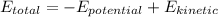
At minimum escape velocity
`E_(total) =0`
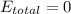
so we have

`E_(kinetic)=E_(potential)`

`(1)/(2) m v_(esc) ^(2) = (G*m*M)/(r) \\ (1)/(2) c ^(2) = (G*M)/(r) \\ r= (2*G*M)/( c^(2) ) \\ r=0.00953m`

Limiting radius is 0.00953m or a bit under 1 cm.