Question

Which expression is equivalent to (1)/(2^(-2)*3^(-2)) ?

Answer 1

B Description is in the picture

Answer 2

`2^2*3^2`

Explanation:

By definition of a negative exponent:
`((a)/(b))^(-x)=((b)/(a))^x=(b^x)/(a^x)`

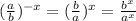
In the last step I simply distributed the exponent, but this actually goes both ways! So we can do:
`(b^x)/(a^x)\implies((b)/(a))^x`

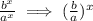
The next thing you need to know is that:
`(ab)^x=a^x*b^x`

and like the previous statement, it works both ways! So this means:
`a^x*b^x\implies (ab)^x`

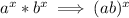
So the denominator of the expression given can be expressed as:

`(2^(-2)*3^(-2))\implies (2*3)^(-2)`

Lastly you need to understand:
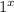`1^x` just simplifies to one, because
`1*1*1*1...\text{ x amount of times}` will stay equal to one. So we can thing of 1 as being raised to some number, even if not explicitly stated.

This helps because we can write one as:
`1^(-2)`

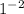
so now we have the expression:

`(1^(-2))/((3*2)^(-2))`

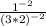
Well this can because now we can "factor" out this exponent just like how it was demonstrated in the beginning

We get the expression:
`((1)/(3*2))^(-2)`

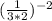
Now we use the definition of a negative exponent, to get the reciprocal giving us the expression

`((3*2)/(1))^(2)`

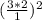
We can now "distribute" this exponent across the division to get

`((3*2)^2)/(1^2)`

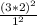
Well 1 squared just simplifies to 1, so it's redundant to write.

This gives us the expression:

`(3*2)^2`

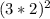
and as stated before we can distribute this across multiplication, and another way to think of it is:

`(3*2)^2\implies (3^2*2^2)\\\\\\text{because } (3*2)^2\implies (3*2)*(3*2)\implies(3*3)*(2*2)`

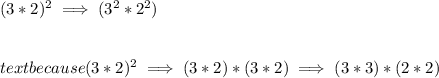
we're just grouping the like terms, so we can write them as an exponent.

So this gives us our final expression:

`3^2*2^2`

or

`2^2*3^2`

which is the option B, since the order in which we multiply does not matter.