Question

The point J is at –9 and point K is at –3. Find the point that divides JK into a 1:2 ratio. The length of JK is 6 units.

Use the number line to complete the statements.

For a ratio of 1:2, divide the length of JK into ? equal parts.

The point that divides JK in a 1:2 ratio is at ?
PLEASE HELP

Answer 1

6

3

-7

Explanation:

Answer 2

For a ratio of 1:2, divide the length of JK into 3 equal parts. The point that divides JK in a 1:2 ratio is at -7.

Explanation:

It is given that point J is at –9 and point K is at –3. The length of JK is 6 units.

The point divided the line segment JK in 1:2. The sum of ratio is

`1+2=3`

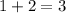
The sum of ratio is 3 it means for a ratio of 1:2, divide the length of JK into 3 equal parts.

The length of JK is 6 units. It means 6 is divided into 3 equal parts.

`(6)/(3)=2`

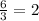
Length of each part is 2 units.

Using section formula.

`x=(mx_2+nx_1)/(m+n)`

`x=(1(-3)+2(-9))/(1+2)`

`x=(-3-18)/(3)`

`x=(-21)/(3)`

`x=-7`

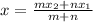
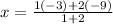
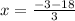
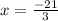
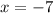
Therefore, the point that divides JK in a 1:2 ratio is at -7.