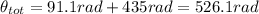Question

At t=0 a grinding wheel has an angular velocity of 20.0 rad/s . it has a constant angular acceleration of 34.0 rad/s2 until a circuit breaker trips at time t = 1.80 s . from then on, it turns through an angle 435 rad as it coasts to a stop at constant angular acceleration. part a through what total angle did the wheel turn between t=0 and the time it stopped?

Answer 1

Let's break the problem into two parts:

1) In the first part, the wheel starts with an angular speed
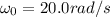
`\omega_0 = 20.0 rad/s` and it rotates with an angular acceleration of
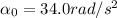
`\alpha_0 = 34.0 rad/s^2` for t=1.80 s. So we can find the total angle covered by the wheel in this part of the motion:

`\theta(t) = \omega_0 t + (1)/(2) \alpha_0 t^2 = 91.1 rad`

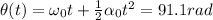
2) Now, the circuit breaker trips, so the wheel starts to decelerate with a certain angular acceleration
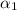
`\alpha_1` (which is negative). During this part of the motion, the wheel covered an angle of
`435 rad`.

3) So, we just need to add the angles the wheel covered in the two parts of the motion:

`\theta _(tot)=91.1 rad+435 rad=526.1 rad`