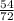A repeating decimal is a decimal that has a digit, or a group of digits, that repeat forever. A terminating decimal, on the other hand, is a decimal that ends. To find which decimals are repeating and which ones are terminal, we are going to simplify our fractions, and express them in decimal form:
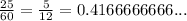
6 is repeating forever, so this is a repeating decimal.
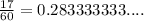
3 is repeating forever, so this is a repeating decimal.
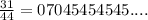
45 is repeating forever, so this is a repeating decimal.
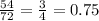
No digit is repeating forever, so this is a terminating decimal.
We can conclude that:
Repeating decimals:
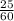
,
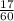
, and
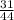 Terminating decimals
Terminating decimals: