Question

Two brothers build a pyramid-shaped fort. If the fort is 8 feet wide at its base, what expression could be used to calculate the height of the fort?

Answer 1

Answer: An expression for the height is: H = (3/64)V

To start with, we will assume that it is a square pyramid, meaning all the sides of the base are 8 feet wide.

Now, we need the formula for the volume of a square pyramid.

V = BH/3

We know the area of the base, it is 8 x 8 = 64, so we can input that.

V = 64H/3 We can multiply by 3/64 to find an expression for the height of the fort.

(3/64)V = H

Answer 2

`h=4tan\alpha`

Explanation:

We know that the base is 8 feet wide. The height of the pyramid is at the middle of this width, which forms a right triangle, with the angles formed by one side of the pyramid and the base.

Now, to find the expression of the height, we can use these given information and the trigonometric reasons. Specifically, we would use the tangent from the trigonometric reasons, because the height is the opposite leg to the angle, and the adjacent leg would be 4. So, the expression would be:

`tan\alpha=(opposite)/(adjacent)\\ tan\alpha=(h)/(4)\\ h=4tan\alpha`

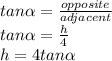
Therefore, the expression is
`h=4tan\alpha`