Question

Is the sequence geometric if so identify the common ratio 1/3, 2/9, 4/27, 8/81, 16/243

Answer 1

yes it is a geometric sequence and the common ratio is 2/3

Answer 2

Yes, the given sequence is geometric and the common ratio is
`(2)/(3)`.

Explanation:

The given sequence is

`(1)/(3),(2)/(9),(4)/(27),(8)/(81),(16)/(243)`

If the ratio of all two consecutive terms are same, then the sequence is called geometric.

`r=(a_(n+1))/(a_n)`

`r_1=((2)/(9))/((1)/(3))=(2)/(3)`

`r_2=((4)/(27))/((2)/(9))=(2)/(3)`

`r_3=((8)/(81))/((4)/(27))=(2)/(3)`

`r_4=((16)/(243))/((8)/(81))=(2)/(3)`

`r_1=r_2=r_3=r_4`

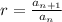
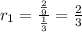
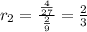
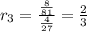
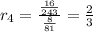
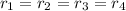
Therefore the given sequence is geometric and the common ratio is
`(2)/(3)`.