Answer:
The nth term for the finite geometric sequence is given by:
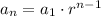 ....[1]
....[1]
where,
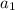 is the first term
is the first term
r is the common ratio of the terms.
As per the statement:
A finite series where
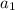 =−3 and r = 1.5.
=−3 and r = 1.5.
Substitute these in [1] we have;
 where, n is the number of terms.
where, n is the number of terms.
To graph the six terms of a finite series.
for n=1

for n = 2

For n = 3

For n = 4
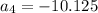
For n = 5
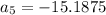
For n= 6
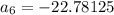
Now,plot these points (1, -3), (2, -4.5), (3, -6.75), (4, -10.125), (5, -15.1875) and (6, -22.78125) on the graph as show below.