Question

How many extraneous solutions does the equation below have? 9 over n squared +1 =n+3 over 4? 0 1 2 3

Answer 1

The answer is 0. While the answer for n is approximately 2.384503, there are no EXTRANEOUS solutions. Again, the answer is a.) 0

Answer 2

Option 1 - There is no extraneous solution i.e. 0.

Explanation:

Given : Expression
`(9)/(n^2+1)=(n+3)/(4)`

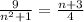
To find : How many extraneous solutions does the equation have ?

Solution :

First we solve to expression to determine the extraneous solution,

`(9)/(n^2+1)=(n+3)/(4)`

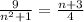
Cross multiply,

`9* 4=(n+3)(n^2+1)`

`36=n^3+n+3n^2+3`

`n^3+3n^2+n-33=0`

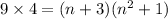
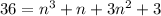
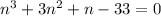
An extraneous solution is defined as a solution, such as that to an equation, that emerges from the process of solving the problem but is not a valid solution to the problem.

The equation form is a cubic function so it has 3 solutions.

Therefore, There is no extraneous solution.