Question

Jake runs either 15 kilometers or 8,500 meters each day.He alternates short and long runs. how many meters farther will he run on his two long run days than on his two short run days?Describe how you found your answer

Answer 1

The problem says that Jake runs either 15 kilometers or 8,500 meters each day and he alternates short and long runs. Then, to solve the problem you must follow the proccedure below:

1. The answer must be expressed in meters, so, you have to convert 15 kilometers to meters:

1 kilometer------1,000 meters
15 kilometers---x=

x=15x1000/1
x=15,000 meters

2. Then, you have:

On his two long run days=(15,000 meters)(2)=30,000 meters

On his two short run days=(8,500 meters)(2)=17,000 meters

3. Finally, you obtain:

30,000 meters-17,000 meters=13,000 meters

How many meters farther will he run on his two long run days than on his two short run days?

The answer is: 13,000 meters

Answer 2

To solve this, the first thing we are going to do in convert 15 km to meters to see which one is the distance of the long runs. To do that we are going to take advantage of the fact that
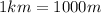
`1km=1000m`, and with this we are going to set up a conversion fraction as follows:

`(15km)( (1000m)/(1km) )=15000km`
We now now that the ditance he covers in his long run days is
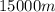
`15000m`, and in his short run is
`8.5km`.
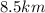
Lets find out the distance he will cover on his two long run days:

`2(15000m)=30000m`
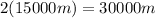
Likewise, lets find the distance he will cover in his short run days:

`2(8500m)=17000m`
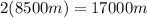
Now, we just need to find the difference between the tow distances:

`30000m-17000m=13000m`

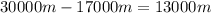
We can conclude that we will run 13,000 meters further in his long runs than in his short runs.