Question

Consider the proof. Given: In △ABC, BD ⊥ AC Prove: the formula for the law of cosines, a2 = b2 + c2 – 2bccos(A) Statement Reason 1. In △ABC, BD ⊥ AC 1. given 2. In △ADB, c2 = x2 + h2 2. Pythagorean thm. 3. In △BDC, a2 = (b – x)2 + h2 3. Pythagorean thm. 4. a2 = b2 – 2bx + x2 + h2 4. prop. of multiplication 5. a2 = b2 – 2bx + c2 5. substitution 6. In △ADB, cos(A) = 6. def. cosine 7. ccos(A) = x 7. mult. prop. of equality 8. a2 = b2 – 2bccos(A) + c2 8. ? 9. a2 = b2 + c2 – 2bccos(A) 9. commutative property What is the missing reason in Step 8?

Answer 1

Solution: The missing reason in Step 8 is substitution of
`x=c\cos (A)`.

Step-by-step explanation:

The given steps are used to prove the formula for law of cosines.

From step 5 it is noticed that our equation is

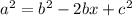`a^2=b^2-2bx+c^2` ..... (1)

From step 7 it is noticed that the value of
`x` is
`c\cos (A)`.

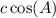So by substituting
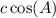`c\cos (A)` for
`x` in equation (1) we get the equation of step 8, i.e.,

`a^2=b^2-2bc\cos (A)+c^2`

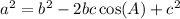
Hence, the missing reason in Step 8 is substitution of
`x=c\cos (A)`.