The electric current is defined as the charge Q that passes a certain point in a time
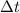
:
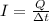
We know the current,
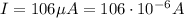
, and the time,
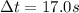
, so the total charge that strikes the target during this time is
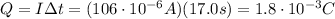
To find the number of proton, we must divide the total charge by the charge of a single proton, which is

:
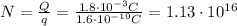
And this is the number of protons that strike the target in 17.0 s.