Answer:
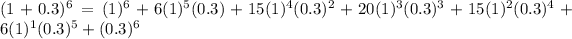
Explanation:
This is an expansion of the expression
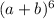 . In general you can expand expressions of this form by a formula known as the binomial theorem. This formula establishes that
. In general you can expand expressions of this form by a formula known as the binomial theorem. This formula establishes that

Where the coeficients
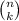 are called binomial coeficients, and can be computed by the formula
are called binomial coeficients, and can be computed by the formula

where
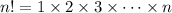 .
.