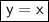Question

What is the equation, in slope-intercept form, of the line that is perpendicular to the line y – 4 = –(x – 6) and passes through the point (−2, −2)?

Answer 1

That line is in point-slope form:


`\sf y-y_1=m(x-x_1)`

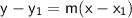
Where 'm' is the slope and (x1, y1) is a point on the line.

Perpendicular lines have opposite slopes. To get the opposite, we take the reciprocal and multiply it by -1.


`\sf -1`

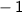
Reciprocal:


`\sf -(1)/(1)\rightarrow -(1)/(1)\rightarrow -1`

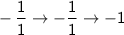
Multiply by -1:


`\sf 1`

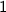
We can plug this slope and the point into point-slope form, and then convert it to slope-intercept form.


`\sf y-y_1=m(x-x_1)`


`\sf y-(-2)=1(x-(-2))`

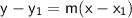
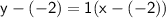
Negatives cancel out:


`\sf y+2=1(x+2)`

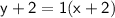
Distribute 1 into the parenthesis:


`\sf y+2=x+2`

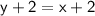
Subtract 2 to both sides:


`\boxed{\sf y=x}`