Question

What else would need to be congruent to show that ABC~XYZ by ASA? given: AB~XY, A~X,

Answer 1

Two triangles are said to be congruent by ASA congrency criterion if the two angles and side between them are equal or congruent.

Refer to the image attached.

To prove:
`\Delta ABC \sim \Delta XYZ`

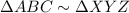
Given:
`AB \cong XY, \angle A \cong \angle X`

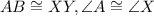
Now, we can observe that the side AB is between the angles A and B and the side XY is between the angles X and Y.

Since it is given that
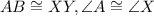`AB \cong XY, \angle A \cong \angle X`, so the condition
`\angle B \cong \angle Y` should be known to shows these triangles congruent.