Question

Angle ABC is formed by two tangents intersecting outside of a circle. If minor arc AC = 110°, what is the measure of angle ABC?

Answer 1

If two tangents intersect at a point outsides the circle, then its angle measure is equal to one-half the difference of its intercepted arcs.

A circle has 360 degrees, so to find the measure of the major arc, subtract 110 from 360 to get 250.

ABC = 1/2(250 - 110)
ABC = 1/2(140)
ABC = 70

The measure of angle ABC is 70 degrees.

Hope this helps =)

Answer 2

Explanation:

Given that two tangents to a circle intersect at a point B outside the circle

A and C are points of contact.

By theorem on circles we have angle ABC is equal to 1/2 the difference of intercepted arcs.

Angle of minor arc =110 and hence major arc
`= 360-110 = 250`

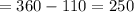
Difference =
`250-110 =140`

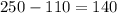
Measure of angle ABC 1/2 of 140 = 70 degrees.