Question

Ashur solves the following system of linear equations.

Which statement about the solution to this system can he say is true?
The values of a and b are both equal to –4.
The values of a and b are both equal to 4.
The value of a is equal to 4, and b is equal to –4.
The value of a is equal to –4, and b is equal to 4.

Answer 1

a. first choice

The values of a and b are both equal to –4.

Answer 2

The given equations that make up the systems are:

-3a+7b=-16...................(Equation 1)

-9a+5b=16...................(Equation 2)

To find the values of a and b we will have to solve the two equations. To solve the equations let us employ the method of elimination.

Let us multiply (Equation 1) by -3. This will give us:

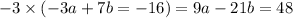`-3* (-3a+7b=-16)=9a-21b=48`...........(Equation 3)

Let us now add (Equation 2) to (Equation 3) to eliminate a. This will give us:

-16b=64

`\therefore b=-4`

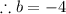
Plugging this value of b in any of the equations we will get the value of a. Let us plug it in (Equation 1). This gives:

`-3(-4)+7b=-16`

`12+7b=-16`

`\therefore b=(-28)/(7)=-4`

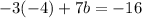
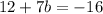
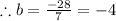
Thus, out of the given options, the first option is the correct one. The values of a and b are both equal to –4.