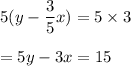Question

Line has an x-intercept of -5 and a y-intercept of 3. Use this information for questions

8 through 10.

8.
Find the slope of the line.



9. Write the equation of the line in slope-intercept form.




10. Write the equation of the line in standard form.

Answer 1

`(1)\;\;\;\text{Slope }= (3)/(5)\\`

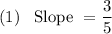
(2) Equation of line in slope-intercept form is

`y = (3)/(5)x + 3`

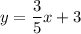
(3) Equation of line in standard form is:

`5y - 3x = 15`

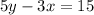
Explanation:

Equation of a line in slope-intercept form is
y = mx + b

where m = slope and b = y-intercept

Slope =
`(y2 - y1)/(x2-x1)`

where (x1, y1) and (x2, y2) are two points on the line.

y2 - y1 is known as the rise
x2 - x1 is known as the run
Slope is also defined as rise/run

We are given x-intercept = -5.
The ex-intercept is the point at which the line crosses the x-axis. At this point the y-value = 0.

So we get one of the points (-5, 0)

We are given y-intercept = 3
The y-intercept is the point at which the line crosses the y-axis. At this point the x-value is 0

So another point on the line is (0, 3)

Knowing this we can compute rise and run
rise = y2 - y1 = 3 - 0 = 3

run = x2 - x1 = 0 - (-5) = 0+5 = 5

`(1)\;\;\;\text{Slope = $(3)/(5)$}`

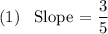
(2) Equation is
y = mx + b where m = slope and b = y-intercept
Slope = 3/5 from (1) and y-intercept = 3

So equation of line in slope-intercept form is

`y = (3)/(5)x + 3`

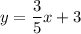
(3) Equation in standard form
In (2) subtract (3/5)x from both sides.

`y - (3)/(5)x = 3`

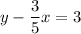
We can multiply both sides by 5 to get

`5(y - (3)/(5)x )= 5 * 3\\\\= 5y - 3x = 15`