Question

SOMEONE HELP ASAPPPPPPP!!! PLEASE

1. A person at ground level measures the angle of elevation to the top of a building to be 74°. If at this point the person is 34 feet away from the base of the building, then how tall is the building? Please round to the tenths place. Please show work/steps and provide units with your answer.

2. In right triangle PQR, P and Q are complementary angles. The value of sin Q is 9/ . What is the value of cos P?
A.9/41
B.41/9
C.40/41
D/41/40

Answer 1

(1) See below for a diagram. Basically, the distance on the ground from the person to the building (34 ft) is adjacent to the angle of elevation (74 degrees) and the height of the building (labeled h in the diagram) is the side opposite the angle. Since we are dealing with opposite and adjacent we use the tangent of the angle and tan = opp/adj

Specifically,
`tan74= (h)/(34)`

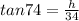
`h=(34)(tan74)`<span></span>

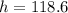
`h=118.6` feet.

Please be sure your calculator is set to degrees (not radians) when you do this problem.

(2) Here since P & Q are complimentary it means that their sum is 90 degrees. Since this is a right triangle that means that the remaining angle (R) must be the right angle. See below for a diagram.

sin = opp/hyp. As the sin Q = 9/41 this means that 9 is the length of the side opposite Q (the side PR) and 41 is the length of the hypotenuse. This makes the remaining side (QR) 40 in length.

cos = adj/hyp. If we focus on angle P the side adjacent (next to) is 9 and the hypotenuse is 41. Thus the cos of P = 9/41.

You could have also realized that if P & Q are complimentary the sin P = cos Q and the cos P = sin Q. We were not asked about tangent but it is also the case that tan P = cot Q and cot P = tan Q.