Question

What is the equation in slope-intercept form of the linear function represented by the table?

Х
y
-5
14
-2
11
1
8
4
5

Answer 1

y = -x + 9

Explanation:

You are given (-5,14), (-2,11), (1,8), and (4,5). Your first objective is to find the slope.

Slope is delta y divided by delta x - the amount of height the graph gains divided by the amount of horizontal length the graph gains. Take any two points and plug it into this:
`(y_(2)-y_(1) )/(x_(2)-x_(1))`.

i.e. (-2,11) and (4,5):
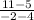`(11-5)/(-2-4)` which is
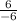`(6)/(-6)`, or -1. Thus, the slope is -1.

We now have y = -1(x) + b, or just y = -x + b.

Next, find the y-intercept. Set the value of x to 0, as the y-intercept is found along the y-axis, which is at the horizontal center of the graph, 0. We now know that the y-intercept is (0,y). To find the y-value, plug one of the coordinate pairs of choice into x and y.

i.e. (-5,14): 14 = (-1)(-5) + b

14 = 5 + b

14 - 5 = 5 + b - 5

9 = b

So, the y-intercept is (0,9).

Finally, the equation is:

y = -x + 9