Question

For the reaction represented by the equation pb(no3)2 + 2ki → pbi2 + 2kno3, how many moles of lead(ii) iodide are produced from 300. g of potassium iodide and an excess of pb(no3)2?

a. 11.0 mol selected:
b. 1.81 mol
c. 3.61 mol
d. 0.904 mol

Answer 1

Answer : The correct option is, (d) 0.904 mole

Explanation : Given,

Mass of potassium iodide = 300 g

Atomic mass of potassium iodide = 166 g /mole

First we have to calculate the moles of potassium iodide.

`\text{Moles of KI}=\frac{\text{Mass of KI}}{\text{Molar mass of KI}}=(300g)/(166g/mole)=1.807mole`

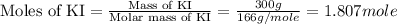
Now we have to calculate the moles of lead(ii) iodide.

The given balanced chemical reaction is,

`Pb(NO_3)_2+2KI\rightarrow PbI_2+2KNO_3`

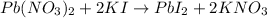
From the given balanced chemical reaction, we conclude that

As, 2 moles of potassium iodide react to give 1 mole of lead(ii) iodide

So, 1.807 moles of potassium iodide react to give
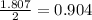`(1.807)/(2)=0.904` mole of lead(ii) iodide

Therefore, the number of moles of lead(ii) iodide produced are, 0.904 mole

Answer 2

Number of moles equals of potassium iodide;

The molar mass of potassium iodide is 166 g/mole

Moles = 300/166

= 1.8072moles

According to the equation;

2 moles of KI produces 1 mole of PbI2 9lead (ii) iodide

Therefore; the number of moles of lead (ii) iodide produced will be;

= 1.8072/2

= 0.9036moles

Thus the number of moles of lead (ii) iodide is 0.904 mole