Answer:
The ladder reaches 142 inches and the base of ladder is 25 inches far from the base of the wall.
Explanation:
Let us draw a diagram for the given situation.
In the attached figure,
AB = the height of wall where the ladder reaches
AC = ladder
BC = distance of base of ladder from the base of the wall
In triangle ABC,

And
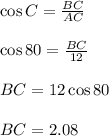
Now, 1 feet = 12 inches
Hence, 11.82 ft = 141.84 inches
and 2.08 feet = 24.96 inches
Therefore, in the nearest inches, we have
The ladder reaches 142 inches and the base of ladder is 25 inches far from the base of the wall.