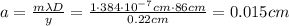Question

Monochromatic light with a wavelength of 384 nm passes through a single slit and falls on a screen 86 cm away. If the distance of the first-order dark band is 0.22 cm from the center of the pattern, what is the width of the slit?

Answer in units of cm.

Answer 1

This is a Fraunhofer single slit experiment, where the light passing through the slit produces an interference pattern on the screen, and where the dark bands (minima of diffraction) are located at a distance of

`y= (m\lambda D)/(a)`
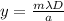
from the center of the pattern. In the formula, m is the order of the minimum,
`\lambda` the wavelenght,
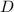
`D` the distance of the screen from the slit and
`a` the width of the slit.

In our problem, the distance of the first-order band (m=1) is
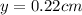
`y=0.22 cm`. The distance of the screen is D=86 cm while the wavelength is
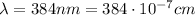
`\lambda = 384 nm=384 \cdot 10^(-7)cm`. Using these data and re-arranging the formula, we can find a, the width of the slit:

`a= (m \lambda D)/(y)= (1 \cdot 384 \cdot 10^(-7)cm \cdot 86 cm)/(0.22 cm)=0.015 cm`