Question

Prove that √2 +√5 is irrational

Answer 1

We have to prove that
`√(2)+√(5)` is irrational. We can prove this statement by contradiction.

Let us assume that
`√(2)+√(5)` is a rational number. Therefore, we can express:

`a=√(2)+√(5)`

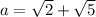
Let us represent this equation as:

`a-√(2)=√(5)`

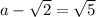
Upon squaring both the sides:

`(a-√(2))^(2)=(√(5))^(2)\\a^(2)+2-2√(2)a=5\\a^(2)-2√(2)a=3\\√(2)=(a^(2)-3)/(2a)`

Since a has been assumed to be rational, therefore,
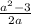`(a^(2)-3)/(2a)` must as well be rational.

But we know that
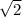`√(2)` is irrational, therefore, from equation
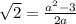`√(2)=(a^(2)-3)/(2a)` the expression
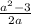`(a^(2)-3)/(2a)` must be irrational, which contradicts with our claim.

Therefore, by contradiction,
`√(2)+√(5)` is irrational.

Answer 2

Explanation:

Let us assume
`\bf √(2)+√(5)` as rational.

`\therefore \: \:\bf √(2)+√(5) = (a)/(b)`

• (where a and b are coprimes)

`\implies\:\:\bf √(5) = (a)/(b) - √(2)`

`\implies\:\:\bf(√(5))^(2) = \bigg( (a)/(b) -√(2)\bigg)^(2)`

• squaring on both sides

`\implies\:\:\bf 5 = (a^(2) )/(b^(2)) - 2 \bigg((a)/(b)\bigg) (√(2)) + (√(2))^(2)`

`\implies\:\:\bf 5 = (a^(2) )/(b^(2)) - 2 √(2) \bigg( (a)/(b) \bigg) + 2`

`\implies\:\:\bf 5-2 = (a^(2))/(b^(2))- 2 √(2) \bigg( (a)/(b) \bigg)`

`\implies\:\:\bf 3 = (a^(2))/(b^(2))- 2 √(2) \bigg( (a)/(b) \bigg)`

`\implies\:\:\bf 2 √(2) \bigg( (a)/(b) \bigg)= (a^(2))/(b^(2)) -3`

`\implies\:\:\bf 2 √(2) \bigg( (a)/(b) \bigg)=(a^(2)-3b^(2))/(b^(2))`

`\implies\:\:\bf 2 √(2) = \bigg( (a^(2)-3b^(2))/(b^(2)) \bigg) \bigg( (b)/(a)\bigg)`

`\implies\:\:\bf √(2) =(a^(2)-3b^(2) )/(2ab)`

• 
  `\bf Irrational \cancel{=} Rational`

`\textsf Hence, our assumption is wrong`

`\therefore \:\:\sf {{\bf{√(2)+√(5)}} is irrational }`

`\mathfrak\purple{{\purple{\bf{H}}}ence\: {\purple{\bf{P}}}roved}`