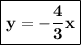Question

Write an equation for the line graphed below.

Answer 1

-4/3x is the correct answer as provided by IceJadeKitsune

I am merely providing an explanation in case you are curious

Explanation:

The equation for a line in slope-intercept form is

y = mx + b

where m is the slope and b the y-intercept

The slope of a line, m can be determined by what is called the rise/run ratio

The process is as follows

1. Take any two convenient points on the line and note their coordinates
2. Let's label the points as (x1, y1) and (x2, y2)
3. The rise is the difference in the y values = y2 - y1 (
4. The run is the difference in the x values = x2 - x1
5. Divide this rise over run and you get the slope, m
6. To get b, find where the line crossed the y-axis and the value of b will be the value of y at that point.
   
   The value of x will be 0 at the y-intercept

From the graph choose points origin (0, 0) which is one point where the line crosses the y axis

From the graph we see that another distinct point where the line passes is at x = -3, y = 4 or the point(-3, 4)

Having got these two points we calculate
rise = 4 - 0 = 4
run = -3 - 0 = -3
Slope m = -4/3

Slope is negative because as y increases, x decreases

So the equation is y = -4/3x + b

Looking at the graph, we see that b = 0 since the graph passes through the origin as correctly stated by IceJadeKitsune


Therefore the equation of the line is
`\boxed{\bold{y = -(4)/(3)x}}`

Answer 2

y=-4/3x

Explanation:

There is no b because it starts at the origin.