Answer:
B. False.
Explanation:
We have been given an image of a quadrilateral. We are asked to determine whether a circle could be circumscribed about the given quadrilateral.
We know that opposite angles of a cyclic quadrilateral are supplementary.
Let us check is this true for our given quadrilateral or not.

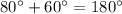
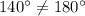
Now let us check other pair of angles.
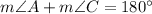

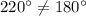
Since opposite angles of our given quadrilateral are not supplementary, therefore, a circle could not be circumscribed about the given quadrilateral.