Answer:
Table 1
Explanation:
We have the function
 .
.
Now, the function g(x) is obtained by reflecting f(x) across y-axis.
i.e. g(x) = f(-x)
i.e.
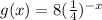
So, substituting the values of x in f(x) or g(x), we will discard some options.
2. For x=0, the value of
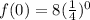 i.e. f(0) = 8.
i.e. f(0) = 8.
As in table 2, f(0) = 0 is given, this is not correct.
3. For x=0, the value of
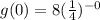 i.e. g(0) = 8.
i.e. g(0) = 8.
As in table 3, g(0) = -8 is given, this is not correct.
4. For x=0, the value of
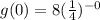 i.e. g(0) = 8.
i.e. g(0) = 8.
As in table 3, g(0) = 0 is given, this is not correct.
Thus, all the tables 2, 3 and 4 do not represent these functions.
Hence, table 1 represents f(x) and g(x) as the values are satisfied in this table.