Answer:

Explanation:
By the given description of trapezoid we get,
The height of the trapezoid h= 6 inches
The base of triangle = 4 inches
The base of rectangle (a)= 8 inches
The bottom base of the trapezoid (b)= 2×base of triangle +base of rectangle
The bottom base of the trapezoid (b)=
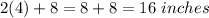
The area of trapezoid is given by :-
