Question

What is the length of ST, to the nearest tenth of a yard?

Answer 1

Let's say that ST is side b
Use the Law of Sines to find side b.
b / sin(B) = a / sin(A)
b / sin(59°) = 7 / sin(79°)
b = (sin(59°) x 7) / sin(79°)
b = 6.112

That is answer B.) 6.1 yards

Hope this helps!

Answer 2

Answer: Second Option is correct.

Explanation:

Since we have given that

ΔSRT is a triangle with measures :

SR = 7 yd

∠T = 79°

∠R = 59°

We need to find the side ST say x.

We will use " Law of Sines " :

`(\sin T)/(SR)=(\sin R)/(ST)\\\\(\sin 79^\circ)/(7)=(\sin 59^\circ)/(x)\\\\0.14=(\sin 59^\circ)/(x)\\\\x=(\sin 59^\circ)/(0.14)\\\\x=6.12\ yd`

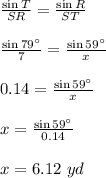
Hence, the length of ST = 6.1 yd.

Therefore, Second Option is correct.