Answer:
(B) DE
Explanation:
Given: Polygon ABCDE is the result of a reflection of polygon LMNOP over the line.
To find: A line segment in the image corresponds to OP in the pre-image.
Solution: It is given that Polygon ABCDE is the result of a reflection of polygon LMNOP over the line.
Also, we know that reflection maps a congruent image, therefore polygon ABCDE is congruent to polygon LMNOP.
And, if two polygons are congruent then their corresponding sides are congruent, thus
LM=AB
MN=BC
NO=CD
OP=DE
AE=LP
Hence, the line segment in the image corresponds to
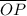 in the pre-image is
in the pre-image is
 .
.
Therefore, option B is correct.